The Road to Reality Study Notes

Each week The Road to Reality Book Club tackles a chapter of Sir Roger Penrose's Epic Tome. We use these meetings as an opportunity to write down the major points to be taken from our reading. Here we attempt to sum up what we believe Penrose was trying to convey and why. The hope is that these community-generated reading notes will benefit people in the future as they go on the same journey.

Other Resources

• The Portal Book Club - We have a weekly group that meets to talk about this book. Come join us in Discord!
• Chronological guide to concepts introduced in TRTR Google Doc
• Book Club Resources in Google Drive

Chapter 1 The Roots of Science

1.1 The quest for the forces that shape the world

Understanding natural processes has been a common pursuit since the dawn of humanity. After many millennia of chaos and frustration, it was discovered that the regular movement of celestial bodies, such as the sun and moon, could be described mathematically. It became apparent that mathematics unlocked deep truths about the universe. Many people in ancient times allowed their imaginations to be carried away by their fascination with the subject, leading to mystical associations with mathematical objects. One famous example from the ancient Greeks is the association between Platonic solids and the basic elementary states of matter.

1.2 Mathematical truth

There was a need to define a more rigorous method for differentiating truth claims. The Greek philosopher Thales of Miletus (c. 625-547 BC) and Pythagoras of Samos (c. 572-497 BC) are considered to be the first to introduce the concept of mathematical proof. Developing a rigorous mathematical framework was central to the development of science. Mathematical proof allowed for much stronger statements to be made about relationships between the arithmetic of numbers and the geometry of physical space.

A mathematical proof is essentially an argument in which one starts from a mathematical statement, which is taken to be true, and using only logical rules arrives at a new mathematical statement. If the mathematician hasn't broken any rules then the new statement is called a theorem. The most fundamental mathematical statements, from which all other proofs are built, are called axioms and their validity is taken the be self-evident. Mathematicians trust that the axioms, on which their theorems depend, are actually true. The Greek philosopher Plato (c.429-347 BC) believed that mathematical proofs referred not to actual physical objects but to certain idealized entities. Physical manifestations of geometric objects could come close to the Platonic world of mathematical forms, but they were always approximations. To Plato the idealized mathematical world of forms was a place of absolute truth, but inaccessible from the physical world.

1.3 Is Plato's mathematical world "real"?

Penrose asks us to consider if the world of mathematics in any sense real. He claims that objective truths are revealed through mathematics and that it is not a subjective matter of opinion. He uses Fermat's last theorem as an point to consider what it would mean for mathematical statements to be subjective. He shows that "the issue is the objectivity of the Fermat assertion itself, not whether anyone’s particular demonstration of it (or of its negation) might happen to be convincing to the mathematical community of any particular time". Penrose introduces a more complicated mathematical notion, the axiom of choice, which has been debated amongst mathematicians. He notes that "questions as to whether some particular proposal for a mathematical entity is or is not to be regarded as having objective existence can be delicate and sometimes technical". Finally he discusses the Mandelbrot set and claims that it exists in a place outside of time and space and was only uncovered by Mandelbrot. Any mathematical notion can be thought of as existing in that place. Penrose invites the reader to reconsider their notions of reality beyond the matter and stuff that makes up the physical world.

For further discussion from Penrose on this topic see Is Mathematics Invented or Discovered?

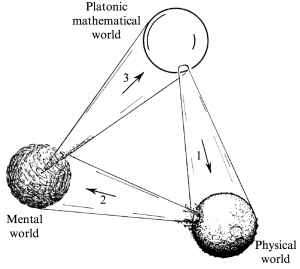
1.4 Three worlds and three deep mysteries

Figure 1.3: Penrose three worlds

Penrose outlines his conception of three worlds:

• The Platonic Mathematical
• The Physical
• The Mental

as show in figure 1.3. Connections between these worlds present a great number of mysteries. The main focus of the book is to explore the connection between mathematics and it's use in describing the physical world. Note that only a small subset of the mathematical world us utilized in describing the physical world. The reason why mathematics can describe the physical world so accurately is unknown. Moving in the counter clockwise direction there is a mysterious connection between the physical world and that of the mind. He believes that there must be some basis of consciousness in physical reality, but it is still unknown. Finally there is the connection between the mental world and mathematics. Penrose believes that there is no mathematical notion beyond our mental construction. He highlights that this figure represents many of his prejudices and might upset some people. Maybe the connections from one world do not fully describe the other, or are incomplete. Penrose believes that not much progress can be made with respect to the mental world until we know much more about the physical world.

1.5 The Good, the True, and the Beautiful

The full conception of Plato's theory of forms was not limited to only mathematical notions. Mathematics was linked to the concept of Truth but Plato was also interested in the absolute idealized forms of Beauty and Good. Beauty plays an important role in many mathematical discoveries and is often used as a guide to the truth. Questions of morality are of less relevance in this context but are critical with respect to the mental world. Moral debates are outside of the scope of this book but must be considered as science and technology progress. Penrose notes that figure 1.3 has purposely been constructed to be paradoxical in the sense that each world is entirely encompassed by the next. He writes "There may be a sense in which the three worlds are not separate at all, but merely reflect, individually, aspects of a deeper truth about the world as a whole of which we have little conception at the present time."

Chapter 2 An ancient theorem and a modern question

• summary

Chapter 3 Kinds of number in the physical world

3.2 The real number system

Natural Numbers:

‘Counting’ numbers from 1 to infinity.

Whole Numbers:

All counting numbers including 0, cannot be a fraction.

Integers:

All natural numbers and their negative counterparts and 0. If and are integers, then their sum , their difference , and their product are all integers (that is, the integers are closed under addition and multiplication), but their quotient may or may not be an integer, depending on whether can be divided by with no remainder.

Rational Numbers:

A number that can be expressed as the ratio a/b of two integers (or whole numbers) a and b, with b non-zero. The decimal expansion is alyas ultimately periodic, at a certain point the infinite sequence of digits consists of some finite sequence repeated indefinitely.

Irrational Numbers:

A number that cannot be expressed as the ratio of two integers. When an irrational number is expressed in decimal notation it never terminates nor repeats.

Quadratic Irrational Numbers

Arise in the solution of a general quadratic equation:

<math>Ax^{2} + Bx + C = 0</math>

With A non-zero, the solutions being (derived from the quadratic formula):

<math>-\frac{B}{2A}\sqrt{\left(\frac{B}{2A}\right)^2}+\frac{C}{A}, \quad -\frac{B}{2A}\sqrt{\left(\frac{B}{2A}\right)^2}-\frac{C}{A}</math>

where, to keep within the realm of real numbers, be must have B2 greater than 4AC. When A, B, and C are integers or rational numbers, and where there is no rational solution to the equation, the solutions are quadratic irrationals.

Real Numbers:

A number in the set of all numbers above that falls on the real number line. It can have any value.

Algebraic Real Numbers:

Any number that is the solution to a polynomial with rational coefficients.

Transcendental Real Numbers

Any number that is not the solution to a polynomial with rational coefficients.

Chapter 4 Magical Complex Numbers

Penrose introduced the complex numbers, extending addition, subtraction, multiplication, and division of the reals to the system obtained by adjoining i, the square root of -1. Polynomial equations can be solved by complex numbers, this property is called algebraic closure and follows from the Fundamental Theorem of Algebra.

Complex numbers can be visualized graphically as a plane, where the horizontal coordinate gives the real coordinate of the number and the vertical coordinate gives the imaginary part. This helps understand the behavior of power series; for example, the power series $$1-x^2+x^4+\cdots$$ converges to the function $$1/(1+x²)$$ only when $$|x|<1$$, despite the fact that the function doesn't seem to have "singular" behavior anywhere on the real line. This is explained by singularities at $$x=i,-i$$.

Finally, the Mandelbrot set is defined as the set of all points $$c$$ in the complex plane so that repeated applications of the transformation mapping $$z$$ to $$z^2+c$$, starting with $$z=0$$, do not escape to infinity.

Chapter 5 Geometry of logarithms, powers, and roots

This is a first pass of main topics in this chapter. This should be expanded.

5.1 Geometry of complex algebra

What addition and multiplication look like geometrically on a complex plane.

• law of addition
• law of multiplication
• addition map
• multiplication map
  • what does multiply by i do? rotate

5.2 The idea of the complex logarithm

Relation between addition and multiplication when introducing exponents.

• $$b^{m+n} = b^m \times b^n$$

5.3 Multiple valuedness, natural logarithms

Different values can arrive at the same value. Rotation brings you back to the same place repeatedly.

• $$e^{i\theta}$$ is helpful notation for understanding rotating
• $$e^{i\theta} = cos \theta + i sin \theta$$
• (Worth looking into Taylor Series, which is related.)

Chapter 6 Real-number calculus

6.1 What makes an honest function?

• Differentiable, Analytic

6.2 Slopes of functions

• Derivative is the slope of the tangent line
• Finding the slope of the tangent line for every point

6.3 Higher derivatives; $$C^\infty$$-smooth functions

• Second derivatives
• Euler would require you to have functions that are $$C^\infty$$-smooth
• Not everything that is $$C^\infty$$-smooth is ok for Euler

6.4 The "Eulerian" notion of a function?

• Physics in trying to understand reality by approximating it.

6.5 The rules of differentiation

• Armed with these few rules (and loads and loads of practice), one can become an "expert at differentiation" without needing to have much in the way of actual understanding of why the rules work!

6.6 Integration

• Fundamental theory of calculus shows integration and differentiation are inverse operations.
• If we integrated then differentiate, we get the same answer back. Non-commutative the other way.