Difference between revisions of "Jones polynomial"
Jump to navigation
Jump to search
(Created page with "From Wikipedia, the free encyclopedia In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, i...") |
|||
| (6 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
<div class="floatright" style="text-align: center"> | |||
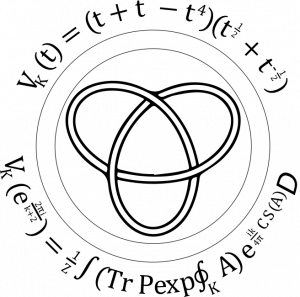
[[File:Jones_polynomial.png|center|class=shadow|300px]] | |||
</div> | |||
'''Vaughan Jones''' (b. 1952) | |||
'''''Jones polynomial''''' 1984 | |||
Resources: | In the mathematical field of [https://en.wikipedia.org/wiki/Knot_theory knot theory], the Jones polynomial is a [https://en.wikipedia.org/wiki/Knot_polynomial knot polynomial] discovered by [https://en.wikipedia.org/wiki/Vaughan_Jones Vaughan Jones] in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a [https://en.wikipedia.org/wiki/Laurent_polynomial Laurent polynomial] in the variable $$ t^{1/2} $$ with integer coefficients. | ||
==Resources:== | |||
*[https://en.wikipedia.org/wiki/Jones_polynomial Jones polynomial] | *[https://en.wikipedia.org/wiki/Jones_polynomial Jones polynomial] | ||
*[https://en.wikipedia.org/wiki/Jones_polynomial#Link_with_Chern%E2%80%93Simons_theory Chern Simons theory] | *[https://en.wikipedia.org/wiki/Jones_polynomial#Link_with_Chern%E2%80%93Simons_theory Chern Simons theory] | ||
Discussion: | ==Discussion:== | ||
[[Category:Pages for Merging]] | |||
Latest revision as of 23:29, 19 October 2022
Vaughan Jones (b. 1952)
Jones polynomial 1984
In the mathematical field of knot theory, the Jones polynomial is a knot polynomial discovered by Vaughan Jones in 1984. Specifically, it is an invariant of an oriented knot or link which assigns to each oriented knot or link a Laurent polynomial in the variable $$ t^{1/2} $$ with integer coefficients.