Difference between revisions of "Fibonacci numbers"
Jump to navigation
Jump to search
(Initial Stub) |
|||
| Line 11: | Line 11: | ||
The beginning of the sequence is thus: | The beginning of the sequence is thus: | ||
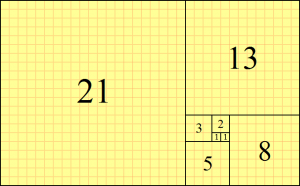
:<math>0,\;1,\;1,\;2,\;3,\;5,\;8,\;13,\;21,\;34,\;55,\;89,\;144,\; \ldots</math> | :<math>0,\;1,\;1,\;2,\;3,\;5,\;8,\;13,\;21,\;34,\;55,\;89,\;144,\; \ldots</math> | ||
The ratio <math> \frac {F_n}{F_{n+1}} \ </math> approaches the [[golden ratio]] as <math>n</math> approaches infinity. | |||
== Resources: == | == Resources: == | ||
Revision as of 08:47, 2 May 2020
In mathematics, the Fibonacci numbers, commonly denoted <math>F_n</math>, form a sequence, called the Fibonacci sequence, such that each number is the sum of the two preceding ones, starting from 0 and 1. That is,
- <math>F_0=0,\quad F_1= 1,</math>
and
- <math>F_n=F_{n-1} + F_{n-2},</math>
for <math>n > 1</math>.
The beginning of the sequence is thus:
- <math>0,\;1,\;1,\;2,\;3,\;5,\;8,\;13,\;21,\;34,\;55,\;89,\;144,\; \ldots</math>
The ratio <math> \frac {F_n}{F_{n+1}} \ </math> approaches the golden ratio as <math>n</math> approaches infinity.